Home Is Where The Wind Blows

Twins, Events and Transformations
I posted this a while ago on Usenet in group sci.physics.relativity.
See the thread: NheA9.17733$Nd.4888@afrodite.telenet-ops.be
(Corrected a few typo's - thanks for the feedback!)

For background information I refer to:
    http://www.math.ucr.edu/home/baez/physics/Relativity/SR/TwinParadox/twin_paradox.html

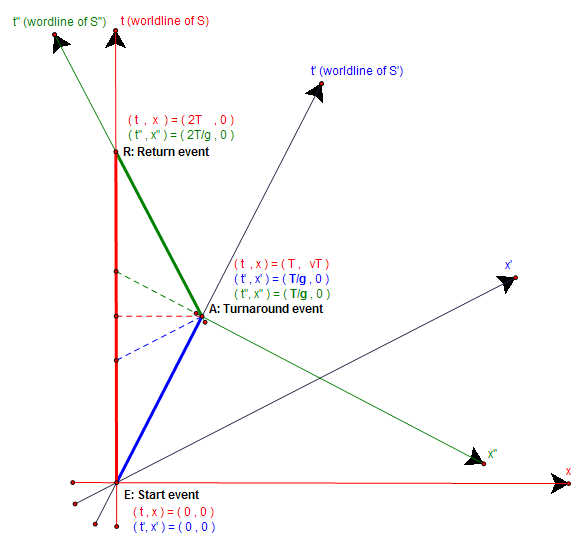
We use 3 inertial reference frames.
        S: The frame of the "stay at home" twin.
        S': The frame of the "outbound part of the trip".
        S": The frame of the "inbound part of the trip".

In none of these frames any form of acceleration is felt.
In order for the travelling twin to make the trip, she must be in frame S'
while going away and in frame S" when coming back. So at the turnaround
event, she must immediately jump from frame S' to frame S" (without getting
hurt!), and she must take over the time her clock is reading to her new
frame. Her clock will then continue ticking until she returns to her twin
brother who remained in his own frame S during the whole process.

Suppose:
        - S uses coordinates (t,x,y,z)
        - S' uses coordinates (t',x',y',z')
        - S" uses coordinates (t",x",y",z") 

   
         \t"(worldline of inbound S")
          \
           \  |t(worldline of S)   /t'(worldline of outbound S')
            \ |                   /              (note added on 20-Nov-2011)
             \| R: Return event  /            Event [p] on clock S, simultaneous
             [R] (t,x) = (2T,0) /                with event [A] according to S'.
              |\ (t",x")=(2T/g,0)             Event [q] on clock S, simultaneous
              | \             /                  with event [A] according to S".
              |  \           /                At event [A], according to the        
              |   \         /                 traveller, clock S "jumps" from  
              |    \       /                  time T-v^2/c^2 to T+v^2/c^2.
 t=2T-T/g^2   |     \     /A: Turnaround event
  =T+v^2/c^2 [q]_    \   /  (t,x)=(T,vT)
              |  ``-._\ /   (t',x')=(T/g,0)            x'_
              |      _[A]._ (t",x")=(T/g,0)        _.-''
 t=T/g^2      | _.-'  /    `--._              __.-'
  =T-v^2/c^2 [p]     /          ``-.__    _.-'
              |     /                _:-.:_
              |    /             _.-'      `--._
              |   /         _.-''               ``-.._ x"
              |  /     __.-'                          `--_
              | /  _.-'
              |/.=                                      
         ----[E]------------------------------------------
              | E: Start event                         x
              |  (t,x) = (0,0)  
              |  (t',x') = (0,0)

1) The Lorentz transformation between S and S'
====================================
Suppose S' recedes from S with velocity v along their x- and x'-axes,
and that they synchronize their clocks at event E with coordinates:
        E: ( t,  x, y,  z ) = ( 0, 0, 0, 0 )
        E: ( t', x', y', z' ) = ( 0, 0, 0, 0 )

Then the Lorentz Transformation between S and S' is given by:
        t' = g(t-vx/c^2)
        x' = g(x-vt)
        y' = y
        z' = z
   where:
        g = 1/sqrt(1-v^2/c^2)   - This is gamma


2) The coordinates of the turnaround event
=================================
Suppose that the outbound twin (now in frame S') has been travelling
during a time T (according to frame S) and decides to turn back to
her brother. Let's call this event A.
According to frame S this event has the coordinates:
        A: ( t, x, y, z ) = ( T, vT, 0, 0 )
To find the coordinates according to the travelling twin who is
in frame S', we apply the Lorentz transformation between S and S'
and find:
        A: ( t', x', y', z' ) = ( T/g, 0, 0, 0 )

We see that the travelling twin's clock shows T/g elapsed time
at this event.
She is now ready to (carefully!) jump on frame S" and return to her
brother.


3) The Lorentz transformation between S and S"
====================================
Frame S" approaches S with velocity v along their x- and x"-axes,
and they synchronize their clocks at event A with coordinates:
        A: ( t,  x,  y,  z  ) = ( T, vT, 0, 0 )
        A: ( t", x", y", z" ) = ( T/g, 0, 0, 0 )

Here we have taken over the already elapsed clock time of the travelling
twin since we are interested in the time the twin's clock will show when
they reunite again.

Then the Lorentz Transformation between S and S" is given by:
        [t"-T/g] = g( [t-T] + v[x-vT]/c^2 )
         x"        = g( [x-vT] + v[t-T] )
         y"        = y
         z"        = z
   where:
        g = 1/sqrt(1-v^2/c^2)

This is the same gamma factor as before since
        v^2 = (-v)^2


4) The coordinates of the return event
=============================
When the twin (now in frame S") has been approaching S during a time T
(again according to frame S), she will reunite with her brother.
Let's call this event R. According to frame S this event has coordinates:
        R: ( t, x, y, z ) = ( 2T, 0, 0, 0 )
Applying the Lorentz transformation between frame S and S", we get:
        R: ( t"-T/g, x", y", z" ) = ( T/g, 0, 0, 0 )
    and thus:
        R: ( t", x", y", z" ) = ( 2T/g, 0, 0, 0 )


5) Conclusion
==========
At this event R both clocks of frames S and S" are at the same place
denoted by (x,y,z) = (x",y",z") = (0,0,0), but
        - the clock of S shows an elapsed time t = 2T
        - the clock of S" shows an elapsed time t" = 2T/g

So if T = 5 years and v = 0.8c, then the stay at home twin will
have aged 10 years (2T) while his travelling twin sister will have
aged 6 years (2T/g).

Dirk Vdm

Hit this to mail me.
(-: Dirk Van de moortel ;-)

   Home Is Where The Wind Blows