Home Is Where The Wind Blows

SR treatment of arbitrarily accelerated motion
 

I posted this a while ago on Usenet in group sci.physics.relativity.
See the thread: a3M1b.82977$F92.8834@afrodite.telenet-ops.be

( V1.4 - Most recent additions and modifications: 5-Aug-2012 )

This is the original ASCII text version. Click here for the MathJax version.

FWIW, I did this as a little exercise a few weeks ago on a vacation
day when it was too hot to do anything else.
Since there are a few active threads on accelerated motion and
whether it can or cannot be treated by special relativity, I decided
to post this for those who might be interested.
Everything is pretty straighforward, so I have not attached numbers
to the equations.
Feel free to point out the typo's and errors.

See also
    http://hermes.physics.adelaide.edu.au/~dkoks/Faq/Relativity/SR/acceleration.html
    http://groups.google.co.uk/groups?&threadm=bi75vd$qbm$1@glue.ucr.edu

Added later:
    http://groups-beta.google.com/group/sci.physics.relativity/msg/dd9168f6ec3220d2
 

Once we know the acceleration that is "felt" by an observer as a
function of his own proper time (called the proper acceleration),
which can be very easily measured, this function can be used to
calculate every aspect of his motion as seen by any interested
inertial observer.

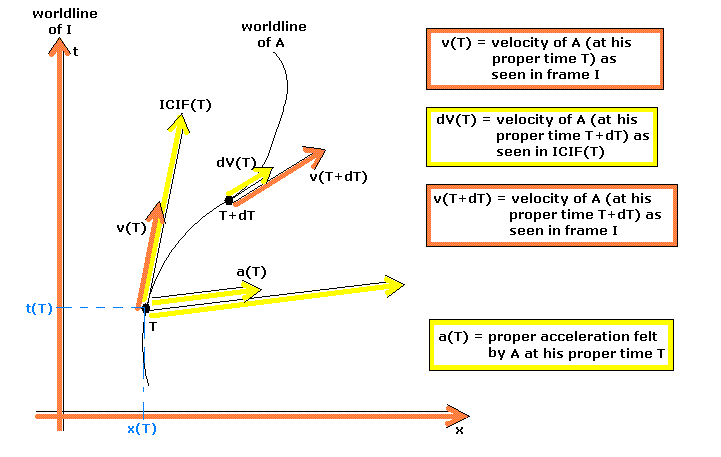
Suppose I is an inertial observer and A is an arbitrarily accelerated
observer that constantly monitors the acceleration he feels as a
function a(T) of his own proper time T (this is 'tau').

At each time T, observer A can write the amount with which his
velocity has changed during a small (infinitesimal) proper time
interval dT during which the acceleration does not change as
        dV(T) = a(T) dT
This change of velocity is to be regarded with respect to the
instantaneously comoving inertial frame at time T.

Observer I can parametrize the worldline of A with the same
proper time T, so he will see infinitesimally consecutive velocities
of A as v(T) and v(T+dT).

Since v(T+dT) is the standard SR composition ('addition') of the
velocities v(T) and dV(T), we can write (using c=1):

        v(T+dT) = [ v(T) + dV(T) ] / [ 1 + v(T) dV(T) ]
 

This part was added for the benefit of Mr. "Mike" aka "Bill Smith" aka "Undeniable" aka "Eleatis". See also the threads "An interesting SR puzzle" and "The Bell Tolls for Dinky-Donkey" You know that SR says that     "When an object P has a relative velocity u w.r.t.      to an inertial frame Q , of which the observer itself      has a relative velocity v w.r.t. an inertial observer R ,     then the object P has a relative velocity          w = (u+v)/(1+u v)     w.r.t. the observer R." Now replace     P => observer A at proper time T+dT     Q => instantaneously comoving inertial frame of observer             at proper time T of observer     R => initial rest frame I     u => dV(T)     v => v(T)     w => v(T+dT) We must do this because it must be valid for *all* values of T, and v(T) becomes quite large, so using the Galilean transformation here would be totally wrong. Together with the fact that dV(T) can be written as         dV(T) = a(T) dT because dT is infinitesimal, that is the very essence of the derivation.

which, using the expression for dV(T), becomes:
        v(T+dT) = [ v(T) + a(T) dT ] / [ 1 + v(T) a(T) dT ]

To calculate dv(T)/dT we use
        [ v(T+dT) - v(T) ] / dT = a(T) [1-v^2(T)] / [ 1 + v(T) a(T) dT ]
Take the limit dT --> 0
        dv(T)/dT = a(T) [ 1 - v^2(T) ]

Rearrange:
        dv(T) / [ 1 - v^2(T) ] = a(T) dT
Integrating between T0 and T and writing v0 = v(T0),
        artanh(v(T)) - artanh(v0) = Int{ T0 to T; a(T') dT' }
and using the abbreviation
        A(T) = Int{ T0 to T; a(T') dT' },
we get
        artanh(v(T)) - artanh(v0) = A(T)

Inverting to
        [ v(T) - v0 ] / [ 1 - v(T) v0 ] = tanh(A(T))
and isolating v(T) gives:
        v(T) = [ v0 + tanh(A(T)) ] / [ 1 + v0 tanh(A(T)) ]
or, written more tersely:
        v(T) = tanh( A(T) + artanh(v0) )
which with v0 = 0 reduces to
        v(T) = tanh(A(T))

Use the standard SR Lorentz transformation (with c=1) between
the frame I and the instantaneously comoving inertial frame of A
at time T:
        dt = gamma(T) ( dT + v(T) dX )
        dx = gamma(T) ( dX + v(T) dT )
Since we are working on the worldline of I where X=0 and thus
dX=0, we get:
        dt/dT = gamma(T)
                = 1 / sqrt( 1-v^2(T) )
                = 1 / sqrt( 1-tanh^2( A(T)+artanh(v0) ) )
                = cosh( A(T)+artanh(v0) )
and
        dx/dT = gamma(T) * v(T)
                 = v(T) / sqrt( 1-v^2(T) )
                 = tanh( A(T)+artanh(v0) ) / sqrt( 1-tanh^2( A(T)+artanh(v0) ) )
                 = sinh( A(T)+artanh(v0) )

Integrate between T0 and T, and writing x0 = x(T0) and t0 = t(T0):
        x(T) = x0 + Int{ T0 to T; sinh( A(T')+artanh(v0) ) dT' }
        t(T) = t0 + Int{ T0 to T; cosh( A(T')+artanh(v0) ) dT' }

If possible, eliminate T to find the equation of the worldline x(t)
of the accelerated observer A in the frame of the inertial
observer I:
        x(t) = ...

If possible, invert the expression for t(T) to find the proper time
of A as a function of the coordinate time t of I:
        T(t) = ...
and use it to find the velocity v(t) as a function of coordinate time t:
        v(t) = tanh( A(T(t))+artanh(v0) )

Summary:
=======
        x and t = coordinates of object as seen in inertial frame.

        a(T) = felt proper acceleration as function of proper time T.
        A(T) = Int{ T0 to T; a(T') dT' }

        v(T) = tanh( A(T)+artanh(v0) )

        dt/dT = cosh( A(T)+artanh(v0) )
        dx/dT = sinh( A(T)+artanh(v0) )

        x(T) = x0 + Int{ T0 to T; sinh( A(T')+artanh(v0) ) dT' }
        t(T) = t0 + Int{ T0 to T; cosh( A(T')+artanh(v0) ) dT' }

        Eliminate T to find the worldline equation x(t)

        Note: These equations are derived in a different way in the article
                 http://arxiv.org/PS_cache/physics/pdf/0411/0411233v1.pdf
                 See equations (3), (4), (5) on page 3.

Special case, when T0 = t0 = x0 = v0 = 0,
===============================
        v(T) = tanh(A(T))

        dt/dT = cosh(A(T))
        dx/dT = sinh(A(T))

        x(T) = Int{ 0 to T; sinh(A(T')) dT' }
        t(T) = Int{ 0 to T; cosh(A(T')) dT' }

Example:
========
The rocket with constant acceleration of the FAQ:
        http://hermes.physics.adelaide.edu.au/~dkoks/Faq/Relativity/SR/rocket.html
Take
        T0 = t0 = x0 = v0 = 0
and
        a(T) = a = constant

So
        A(T) = Int{ 0 to T; a(T') dT' }
               = a T
So
        v(T) = tanh(A(T))
               = tanh(a T)
and
        x(T) = Int{ 0 to T; sinh(a T') dT' }
               = 1/a * ( cosh(a T) - 1 )
and
        t(T) = Int{ 0 to T; cosh(a T') dT' }
               = 1/a * sinh(a T)

Eliminate T:
        (x+1/a)^2 - t^2 = 1/a^2
giving the hyperbola
        x(t) = 1/a [ sqrt( 1 + (a t)^2 ) - 1 ]

Proper time as a function of coordinate time:
        T(t) = 1/a * arsinh(a t)
so
        v(t) = tanh( a T(t) )
              = tanh( arsinh(a t) )
              = a t / sqrt[ 1 + (a t)^2 ]

Re-introduce c and we find as functions of proper time T:
         v(T) = c tanh(a T/c)
         gamma(T) = cosh(a T/c)
         x(T) = c^2/a [ cosh(a T/c) - 1 ]
         t(T) = c/a sinh(a T/c)
and as functions of coordinate time t:
         v(t) = a t / sqrt( 1 + (a t/c)^2 )
         gamma(t) = sqrt( 1 + (a t/c)^2 )
         x(t) = c^2/a ( sqrt( 1 + (a t/c)^2 ) -1 )        (the hyperbola)
         T(t) = c/a arsinh(a t/c)
which are the equations of the FAQ entry.

[ added on 9-Jul-2009 ]
Furthermore we have the momentum given by
        p(t) = m gamma(t) v(t)
              = m a t       (linear)
and the force
        F(t) = dp/dt(t)
              = m a        (constant)

Dirk Vdm

Putting c = 1 and using accent notation for the derivative w.r.t. proper time d/dT:

Velocity 4-vector V = ( t', x', 0, 0 ).
Acceleration 4-vector V' = ( t'', x'', 0, 0 )
with unknown functions t(T) and x(T).

You have V.V = 1, giving
        t'^2 - x'^2 = 1

Since V'.V' is invariant, it must have the value it has in the comoving
inertial frame, giving
        t''^2 - x''^2 = 0 - a^2

These 2 differential equations are pretty common and easily solved:

        t(T) = 1/a sinh( a T )
        x(T) = 1/a cosh( a T ) - 1/a    [ to make x(0) = 0 ]

from which you can eliminate the proper time T and find the hyperbola

        x(t) = 1/a ( sqrt( 1 + (a t)^2 ) -1 )
 

For an "interesting" comment, see also 1158564121.012424.39150@m73g2000cwd.googlegroups.com

Hit this to mail me.
(-: Dirk Van de moortel ;-)

   Home Is Where The Wind Blows